Table of Contents

Introduction:
What is Momentum?
Conservation of Linear Momentum
What are the collisions?
- How exactly does the Collisions happen
Types of Collisions
Applications in daily life:
Conclusions:

Collisions and Momentum

Darshan M.
April 11, 2023

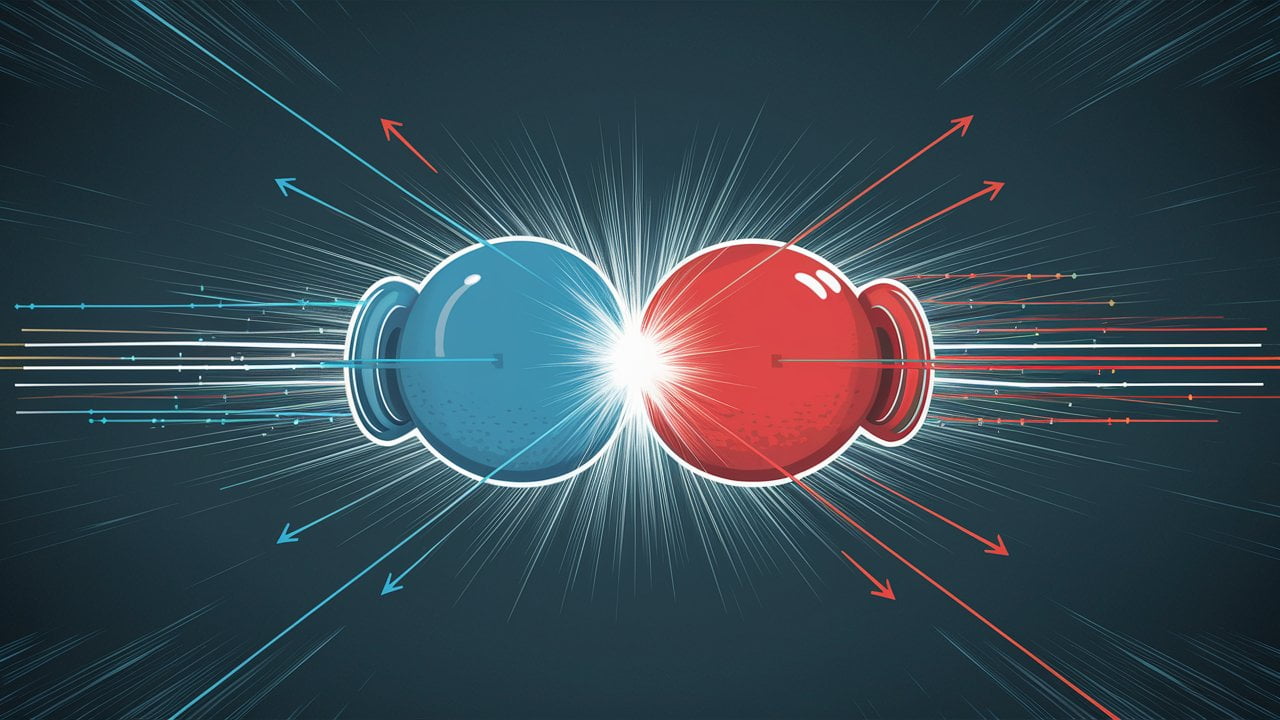
This topic is covered in Physics honors, AP Physics 1 and AP Physics C Mechanics.

Table of Contents

Introduction:
What is Momentum?
Conservation of Linear Momentum
What are the collisions?
- How exactly does the Collisions happen
Types of Collisions
Applications in daily life:
Conclusions:

Introduction:

Have you ever wondered:

How do air particles transmit sound wave energy to your ears?
How do boxing gloves prevent your fist from getting injured during boxing?
Why does a player pull his hands back slightly while catching the ball?

And many more incidents like these.

After reading this blog, you will not only be able to understand the reason behind these incidents, but you will also be able to share the reason behind these incidents with others.

In this blog, we will cover the following topics-

What is Momentum?
Conservation of Linear Momentum
What are the collisions?
Types of collisions
Real World Examples
Applications in various fields
Conclusion

What is Momentum?

Suppose there is a particle of mass m and acceleration

$\vec{a}$

From Newton’s second law of motion, we can write that

$\Sigma \vec{F}=m\vec{a}....(i)$

Where

$\Sigma \vec{F}$

is the resultant force applied on the particle.

Since

$\vec{a}$

is the rate of change of velocity of the particles so equation (i) can be written as-

$\Sigma \vec{F}=m\frac{d\vec{v}}{dt}=\frac{d}{dt}(m\vec{v})...(ii)$

In differential calculus, we read that a constant value can be written in the derivative and mass m of the particle is a constant value. That’s why we can write it in the derivative.

Now the entire term

$m\vec{v}$

inside the derivative is called the Momentum or linear Momentum of this particle. Its plural is momenta. It is represented by

$\vec{P}$

, which is a vector quantity whose direction is along the velocity of the particle. Its SI unit is kilogram meter per second and we can write the following expression-

$\vec{P}=m\vec{v}....(iii)$

So, from equations (ii) and (iii), we can write

$\Sigma \vec{F}=\frac{d\vec{P}}{dt}....(iv)$

This expression says,

“The resultant force on an accelerating particle is equal to the rate of change of momentum of that particle.”

Equation (iv), not equation (I), is the form in which Newton had originally published his second law of motion.

You can feel the concept of Momentum by considering an example as follows-

Suppose there is a truck of mass 345 kilograms moving uniformly with a velocity 20 meter per second, and there is a bullet of mass 0.02 kilogram, which is moving uniformly with a velocity of 3,45,000 metres per second.

The direction of the motion of the bullet and truck is the same.

The Momentum of bullet =the Momentum of truck =6,900 kilogram meter per second

So, in this way, you have to apply the same force {Remember equation (ii)} on them to stop their motion in the same time interval.

Conservation of Linear Momentum

Suppose two astronauts are floating freely in the zero-gravity environment of outer space.

Now think of these astronauts as two particles. Each particle exerts a force on another particle according to Newton’s third law of motion, which are same in magnitude but opposite in direction.

$\vec{F}_{BA}$

is force applied by particle A on particle B and

$\vec{F}_{AB}$

is the force applied by particle B on particle A.

Since,

$\vec{F}_{AB}=-\vec{F}_{BA}$

So,

$\vec{F}_{AB}+\vec{F}_{BA}=0.....(v)$

The forces

$\vec{F}_{AB}$

and

$\vec{F}_{BA}$

which are applied by the particles of the system on each other are called “internal forces,” and The force exerted on a system by an object located outside the system is called an “external force.”

There are no external forces on the system we are dealing with, hence this type of system is called an “isolated system” which is not under any external force, or the resultant of the external forces on the system is zero.

Equation (v) can be written in the form of a summation of rate of change of momenta of the particles as follows-

$\frac{d\vec{P}_{AB}}{dt}+\frac{d\vec{P}_{BA}}{dt}=0$

$\frac{d}{dt}(\vec{P}_{AB}+\vec{P}_{BA})=0...(vi)$

The total Momentum of the system is equal to the vector sum of the Momentum of individual particles of the system,so

$\vec{P}=\vec{P}_{AB}+\vec{P}_{BA}....(vii)$

It means from equations (vi) and (vii), we can write

$\frac{d\vec{P}}{dt}=0$

Hence, the rate of change of total Momentum of the system is zero, which is constant, even though the individual momenta of the particles can be changed.

If there are some external forces, then their resultant can be put in the left side of equation (v) but if there are no external forces, the equation will be as it is. In this way we can say,

“If the vector sum of the external forces on a system is zero then the total momentum of the system is a constant”

This statement is called the conservation of linear Momentum because the total Momentum of the system is conserved.

Similarly, if we have a system consisting n particles and the momenta of these particles are

$\vec{P_1},\vec{P_2},\vec{P_3}......\vec{P_n}$

then the total Momentum of the system will be-

$\vec{P}=\vec{P_1}+\vec{P_2}+\vec{P_3}+.......+\vec{P_n}.....(viii)$

And again the rate of change of total Momentum of the system due to each pair of action-reaction internal force is zero.

Hence the conservation of linear Momentum is still valid.

Note: Since we have used Newton’s second law of motion to derive the law of conservation of linear Momentum so it is important to note that it is valid only in the inertial frame of reference.

What are the collisions?

“Collision” is a word that we use in our daily life to describe the interaction of two or more vehicles that causes damage to the vehicles.

But in physics, “collision” is a term that is used when there is a strong force between any two bodies for a very short period of time (in which they are in contact). This type of force is called an impulsive force.

This collision can happen between two marble balls, between two vehicles, between two air particles and also between two planets or other celestial bodies.

How exactly does the Collisions happen

Consider two elastic balls. They are moving in the same direction with different velocities so at some point in time, they will come in contact with each other and they will start to exert force on each other (Newton’s third law of motion), which results in their deformation.

As the balls deform, their internal reaction force opposes this deformation, and at some point, the internal resistive force becomes equal to the force exerted by these balls on each other and the balls don’t deform any more.

In the deformed state, some part of the kinetic energy of these balls is stored in the form of potential energy into them. Now due to the increase in the magnitude of the internal resistive force, the balls start to gain their initial shape.

When two balls completely return to their original shape after a collision, then it is called a perfectly elastic collision. In this type of collision, during deformation, the total kinetic energy of the system is conserved in the form of elastic potential energy of the balls and again converted into kinetic energy after they collide. It means the kinetic energy of the balls before the collision is equal to the kinetic energy after the collision.

If they partially recover their original shape after collision, then it is called Inelastic collision. In this case, some amount of their kinetic energy is lost . This lost kinetic energy is stored in the form of elastic potential energy of deformed part of the balls and can’t be completely converted into kinetic energy again; only some amount is converted.

After the collision, if there is no recovery at all, then it is called a perfectly inelastic collision. In this case, lost kinetic energy is entirely converted in potential energy of the deformed parts forever and no amount of it can be converted back into kinetic energy. In this type of collision, bodies stick together.

Types of Collisions

There are broadly two kinds of collisions-

Perfectly elastic collision
Inelastic collisions
Elastic collision

1. Elastic collisions:

In this type of collision, the linear Momentum and kinetic energy of the system remain constant.

Now consider two billiard balls A and B of mass

$m_A$

$and$

$m_B$

respectively.

Let their velocities before collision to be

$v_{A1}$

and

$v_{B1}$

respectively and their velocities after the collision to be

$v_{A2}$

and

$v_{B2}$

respectively.

Since kinetic energy and linear Momentum of the system remain constant so

Initial kinetic energy = Final kinetic energy

$and$

And initial Momentum = final Momentum

$Or$

$\frac{1}{2}m_Av_{A1}^2+\frac{1}{2}m_Bv_{B1}^2=\frac{1}{2}m_Av_{A2}^2+\frac{1}{2}m_Bv_{B2}^2.....(ix)$

$and$

$m_Av_{A1}+m_Bv_{B1}=m_Av_{A2}+m_Bv_{B2}.....(x)$

Now after solving equations (ix) and (x) we can calculate the final velocities

$v_{A2}$

and

$v_{B2}$

(after the collision) for billiard balls.

Perfectly elastic collision in one dimension-

If the billiards balls mentioned above are moving along the same direction before the elastic collision and along opposite directions after the elastic collision, then this type of collision is called Head on Collisions or elastic collision in one dimension.

Let

$v_{A1},v_{A2},v_{B1}$

$and$

$v_{B2}$

be the velocities along the X-Axis. Now we will attempt to calculate a general solution of equations (ix) and (x) in which

$v_{A2}$

and

$v_{B2}$

will be related to mass and initial velocities of balls.

Equation (ix) can be written as-

$m_A(v_{A1}^2-v_{A2}^2)=m_B(v_{B2}^2-v_{B1}^2).....(xi)$

Equation (x) can be written as-

$m_A(v_{A1}-v_{A2})=m_B(v_{B2}-v_{B1})....(xii)$

Now divide equation (xi) by (xii) then we will get-

$v_{A1}-v_{B1}=v_{B2}-v_{A2}.....(xiii)$

Here

$(v_{A1}-v_{B1})$

is the rate at which the separation between the balls decreases and

$(v_{B2}-v_{B1})$

is the rate at which their separation increases.

So,

The velocity of separation = the velocity of approaching….(xiv)

Multiply equation (xiii) to

$m_A$

and subtract from equation (x), we will get-

$v_{A2}=\frac{(m_A-m_B)}{m_A+m_B}v_{A1}+\frac{2m_B}{m_A+m_B}v_{B1}....(xv)$

Now multiply equation (xiii) to

$m_B$

and again subtract it with equation (x) then we will get-

$v_{B2}=\frac{2m_Av_{A1}}{m_A+m_B}-\frac{(m_A-m_B)}{m_A+m_B}v_{B1}......(xvi)$

In this way ,we solved the equations (x), and (xiii), and equation (xv) and (xvi) are their solutions.

2. Inelastic collisions:

In this type of collision, the linear Momentum is conserved however and kinetic energy of the system is not conserved.

3. Perfectly Inelastic collision

In this type of collision, when two balls collide, they stick together like balls made of wet clay. It means they have different velocities before the collision but same velocity after the collision.

Let the mass of two elastic balls to be

$m_A$

$and$

$m_B$

and their velocities to be

$v_A$

$and$

$v_B$

respectively. If the common velocity of the balls after the collision is V then

$m_Av_A+m_Bv_B=(m_A+m_B)V$

$V=\frac{m_Av_A+m_Bv_B}{m_A+m_B}....(xvii)$

We know that in perfectly inelastic collisions, the kinetic energy of the system is lost by some amount so if ∆k represent the loss in kinetic energy of the system then

$∆k=\frac{1}{2}[m_Av_A^2+m_Bv_B^2-(m_A+m_B)V^2]$

On putting the value of V from equation (xvii), we will get

$∆k=\frac{m_Am_B(v_A-v_B)^2}{2(m_A+m_B)}$

Real-world examples:

When a sound wave collides with your eardrum, it transfers its Momentum to it. This causes the eardrum to vibrate, which in turn sets the tiny bones in your inner ear in motion. These bones amplify the sound wave and transmit it to the cochlea, which converts the vibrations into electrical signals that are sent to the brain.

Boxing gloves increase the time of impact during a punch, decreasing the force and pressure on the fist, which reduces the likelihood of injury. This is due to the principles of Momentum and collision

When a player pulls their palm slightly behind while catching a ball, it creates a backward force or Momentum that helps to reduce the ball’s speed upon impact. This decrease in speed reduces the force of the collision, making it easier for the player to hold onto the ball.

Newton’s cradle demonstrates the conservation of Momentum and elastic collisions using a series of suspended balls that transfer Momentum from one ball to the next when struck, and the balls swing back and forth until friction causes them to stop.

Applications in daily life:

Car accidents – understanding the principles of Momentum can help us determine the severity of a car accident and the damage caused to the vehicles and passengers involved.

Sports – Momentum plays a crucial role in various sports, such as baseball, basketball, and football. Athletes use the principle of Momentum to optimize their performance and achieve their goals.

Transportation systems – the safety features in cars, such as airbags and crumple zones, are designed to reduce the Momentum of the vehicle and protect passengers from serious injuries during accidents.

Engineering and design – understanding the principles of Momentum is essential in designing efficient machines and structures that can withstand the forces of impact.

Robotics – robots that are designed to navigate through different environments require a thorough understanding of Momentum and collision to avoid obstacles and navigate safely.

Conclusions:

The concept of collision and Momentum is a fundamental aspect of physics that has a significant impact on our daily lives. It has a wide range of applications, from designing safer cars and more efficient machines to improving sports performance and preventing injuries in accidents. Understanding the principles of Momentum can help us make better decisions in various aspects of our lives and improve our overall quality of life. As we continue to explore and apply these principles, we can look forward to a safer, more efficient, and more innovative future.

For Further insights, you can access to the following practice problems: Collisions and Momentum problems

Do you know that collisions and momentum questions find its application in Conservation of Energy? Read this blog Conservation of Mechanical energy

About Colin Phillips

Lorem ipsum dolor amet, conse cetur adipisicing elit, sed do eiusmod tempor incididunt labore et dolore

Collisions and Momentum

Introduction:

What is Momentum?

Conservation of Linear Momentum

What are the collisions?

How exactly does the Collisions happen

Types of Collisions

1. Elastic collisions:

Perfectly elastic collision in one dimension-

2. Inelastic collisions:

3. Perfectly Inelastic collision

Applications in daily life:

Conclusions:

Share

About Colin Phillips

Quick Links

Services

Others

Contact us

Subjects