- Introduction
- An Overview of AP Calculus BC
- Similarities Between AP Calculus AB and BC
- Differences Between AP Calculus AB and BC
- Choosing Between AP Calculus AB and BC
- College Credit and Placement: AB vs. BC
- Preparing for the AP Calculus Exams
- Conclusion: Choosing Your Path in AP Calculus
- Frequently Asked Questions (FRQs) :
Introduction
This blog aims to help you navigate the decision between AP Calculus AB and AP Calculus BC by providing a comprehensive comparison of the two courses. We will explore the curriculum, ideal student profiles, college credit implications, and strategies for success. By the end, you’ll have a clearer understanding of which path aligns best with your academic goals and strengths.
Whether you’re a student deciding which course to take or a parent or educator guiding someone through this choice, this guide will offer valuable insights to make an informed decision.
What You Need to Know About AP Calculus AB
Overview of the AP Calculus AB Curriculum
AP Calculus AB covers the equivalent of a first-semester college calculus course. The curriculum is structured around three main topics:
1. Limits and Continuity
- Understanding the concept of a limit
- Evaluating limits analytically
- Exploring continuity and discontinuities
2. Differentiation
- Defining the derivative and interpreting it as a rate of change
- Techniques of differentiation, including the power, product, quotient, and chain rules
- Applications of derivatives, such as motion, optimization, and curve sketching
3. Integration
- Understanding antiderivatives and indefinite integrals
- The Fundamental Theorem of Calculus
- Techniques of integration, including substitution
- Applications of integration, such as calculating areas under curves and solving problems involving accumulation
Key Topics Covered in AP Calculus AB
The course is structured to provide students with a comprehensive understanding of the following key topics:
- Limits and Asymptotic Behavior: Students learn to calculate limits and understand their significance in calculus.
- Derivatives: The course emphasizes both the theoretical underpinnings and practical applications of derivatives.
- Applications of Derivatives: Students use derivatives to solve problems involving motion, growth, and optimization.
- Integrals: The course covers both definite and indefinite integrals and their applications.
- Fundamental Theorem of Calculus: A critical component that connects differentiation and integration.
Check this out to know more details about AP Calculus AB course.
Ideal Student Profile for AP Calculus AB
AP Calculus AB is suitable for students who:
- Have a strong foundation in algebra, geometry, and precalculus concepts
- Are comfortable with mathematical reasoning and problem-solving
- Are interested in fields such as science, technology, engineering, or mathematics (STEM)
- Prefer a paced introduction to calculus concepts, allowing for a deep understanding of fundamental principles
NOTE: To know more about the AP Precalculus topics, you can explore our detailed blog
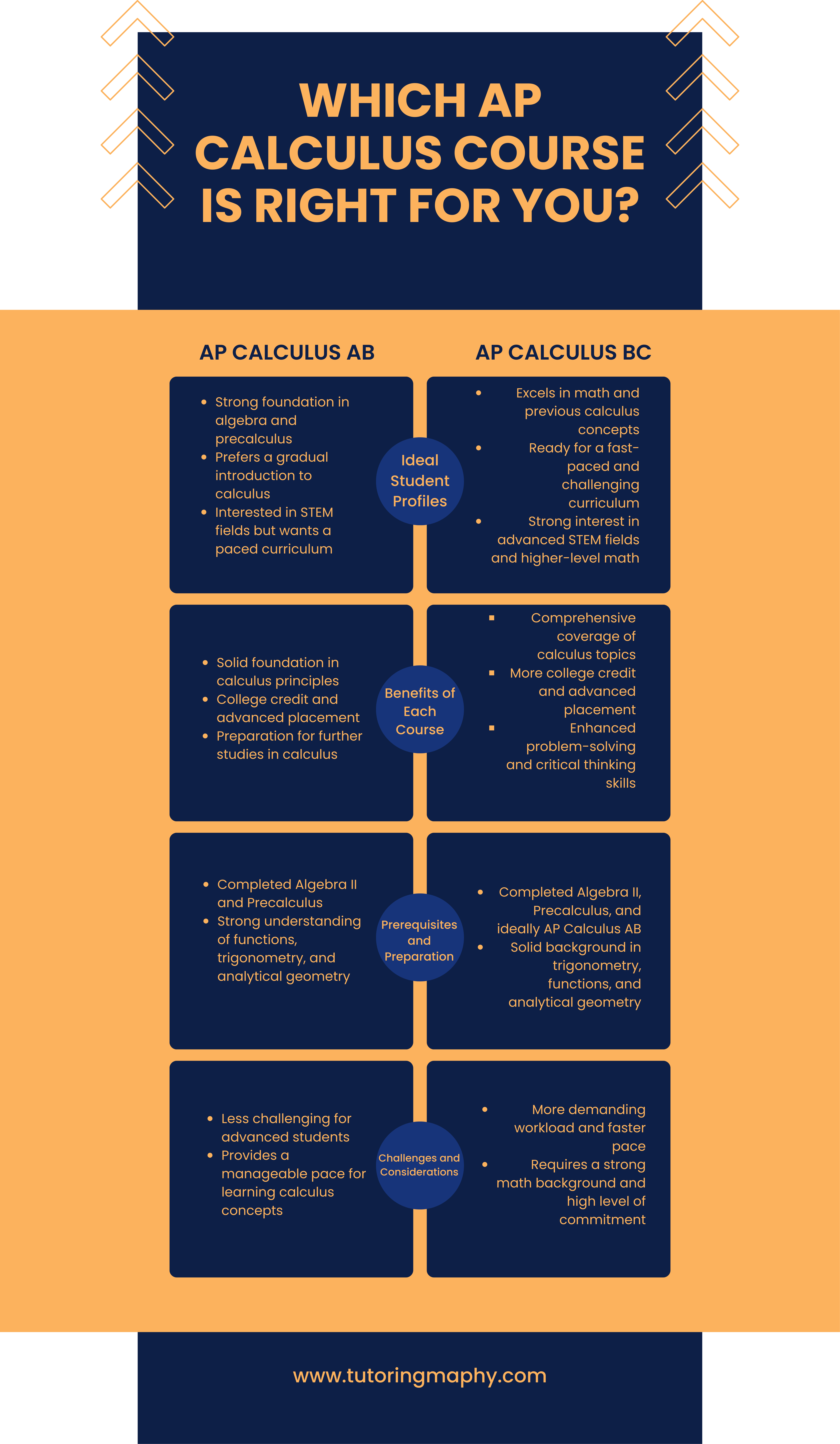
Prerequisites and Preparation
To succeed in AP Calculus AB, students should have completed courses in Algebra II and Precalculus/ AP Precalculus. These courses provide the necessary background in functions, trigonometry, and analytical geometry, which are essential for understanding calculus.
Benefits of Taking AP Calculus AB
- College Credit: Many colleges and universities offer credit or advanced placement for students who score well on the AP Calculus AB exam, potentially saving time and money in college.
If you are interested to know more about credits, checkout How are AP and College credits related?
- Preparation for Advanced Studies: The course provides a strong foundation for future studies in calculus and other higher-level mathematics courses.
- Problem-Solving Skills: Students develop critical thinking and problem-solving skills that are valuable in a wide range of academic and career paths.
An Overview of AP Calculus BC
Overview of the AP Calculus BC Curriculum
1. Limits and Continuity
- Understanding limits and their properties
- Exploring continuity and types of discontinuities
2. Differentiation
- Techniques of differentiation, including advanced methods
- Applications of derivatives in various contexts
3. Integration
- Definite and indefinite integrals
- Advanced integration techniques such as integration by parts and partial fractions
- Applications of integrals, including volume and surface area calculations
4. Polynomial Approximations and Series
- Taylor and Maclaurin series
- Convergence tests for series
- Representing functions as power series
5. Parametric, Polar, and Vector Functions
- Calculus with parametric equations
- Polar coordinates and their applications
- Vector-valued functions and their derivatives and integrals
Key Topics Covered in AP Calculus BC
In addition to the topics covered in AP Calculus AB, AP Calculus BC includes:
- Sequences and Series: Understanding and working with sequences, infinite series, and convergence tests.
- Taylor and Maclaurin Series: Using these series to approximate functions.
- Advanced Integration Techniques: Including integration by parts, partial fractions, and improper integrals.
- Parametric and Polar Functions: Calculus applied to parametric curves and polar coordinates.
- Vector Functions: Introducing vector-valued functions and their applications.
Check this out to know more details about AP Calculus BC course.
Ideal Student Profile for AP Calculus BC
AP Calculus BC is suitable for students who:
- Have a strong foundation in algebra, geometry, precalculus, and introductory calculus concepts
- Are highly motivated and capable of handling a fast-paced and challenging curriculum
- Have a keen interest in mathematics and its applications in various fields
- Are considering a major in mathematics, engineering, physics, computer science, or other STEM fields
Prerequisites and Preparation
To excel in AP Calculus BC, students should have completed courses in Algebra II and Precalculus, and ideally have a solid understanding of the material covered in AP Calculus AB. A strong background in trigonometry, functions, and analytical geometry is essential.
Benefits of Taking AP Calculus BC
- College Credit: Many colleges and universities offer credit or advanced placement for students who perform well on the AP Calculus BC exam, often equivalent to two semesters of college calculus.
- Advanced Preparation: The course provides a comprehensive foundation for further studies in higher-level mathematics and related fields.
- Enhanced Problem-Solving Skills: Students develop advanced critical thinking and problem-solving abilities that are valuable in various academic and professional contexts.
Similarities Between AP Calculus AB and BC
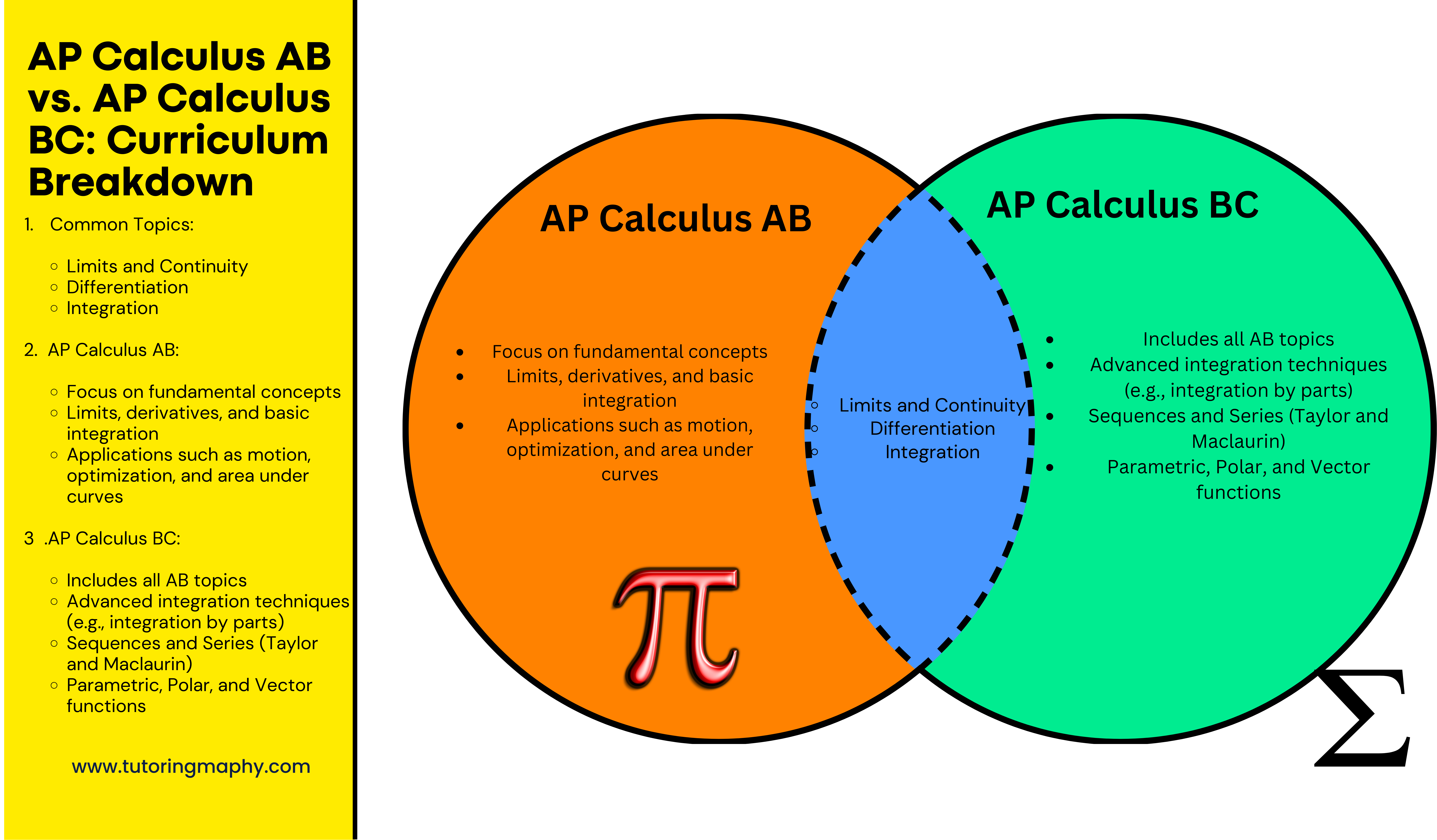
Shared Content and Concepts
Both AP Calculus AB and BC cover fundamental calculus topics, ensuring that students develop a strong understanding of the basics before moving on to more complex material. These shared topics include:
1. Limits and Continuity
- Both courses introduce the concept of limits, teaching students how to evaluate limits analytically and understand their significance in calculus.
- Continuity and different types of discontinuities are covered, providing a basis for understanding the behavior of functions.
2. Differentiation
- The definition and interpretation of the derivative as a rate of change are fundamental in both courses.
- Techniques of differentiation, including the power, product, quotient, and chain rules, are taught in both AB and BC.
- Applications of derivatives, such as motion problems, optimization, and curve sketching, are also common to both courses.
3. Integration
- Both courses cover antiderivatives and indefinite integrals, providing a foundation for more advanced integration techniques.
- The Fundamental Theorem of Calculus is a central topic in both AB and BC, connecting differentiation and integration.
- Applications of integration, such as calculating areas under curves and solving accumulation problems, are explored in both courses.
Similar Skills Developed
Students in both AP Calculus AB and BC develop critical thinking and problem-solving skills through rigorous coursework and challenging problem sets. These skills include:
- Analytical Thinking: Students learn to approach complex problems methodically, breaking them down into manageable parts and applying appropriate calculus techniques.
- Conceptual Understanding: Both courses emphasize a deep understanding of calculus concepts, ensuring that students can apply these concepts in various contexts.
- Mathematical Communication: Students are trained to communicate their mathematical reasoning clearly and accurately, both in written form and verbally
Exam Format and Structure
The AP Calculus AB and AP Calculus BC exams share a similar structure, designed to test students’ understanding and application of calculus concepts. Both exams include:
- Multiple-Choice Questions: These questions assess a broad range of topics and require students to choose the correct answer from several options.
- Free-Response Questions: These questions require students to show their work and explain their reasoning, demonstrating a deeper understanding of the material. To know more about AP Calculus BC FRQs, click here
- Graphing Calculator Use: Both exams allow the use of graphing calculators for certain sections, testing students’ ability to apply technology to solve calculus problems.
Preparation for Future Studies
Both AP Calculus AB and BC prepare students for further studies in mathematics, science, engineering, and related fields. By completing either course, students gain a solid foundation in calculus that will support their academic and career pursuits.
Differences Between AP Calculus AB and BC
Additional Topics in AP Calculus BC
AP Calculus BC covers all the material in AP Calculus AB, but it extends further into more advanced topics. Some of the additional topics in AP Calculus BC include:
1. Sequences and Series
- Introduction to sequences and infinite series
- Tests for convergence, including the Ratio Test and the Integral Test
- Power series and the representation of functions as series
- Taylor and Maclaurin series for function approximation
2. Parametric, Polar, and Vector Functions
- Calculus with parametric equations, including derivatives and integrals
- Polar coordinates: graphing and calculating areas and lengths in polar form
- Vector-valued functions: understanding and computing derivatives and integrals of vector functions
3. Advanced Integration Techniques
- Integration by parts
- Partial fractions
- Improper integrals
Differences in Depth and Complexity
AP Calculus BC delves deeper into the topics it shares with AP Calculus AB, providing a more thorough and complex understanding of calculus concepts. The additional material and increased complexity mean that AP Calculus BC is more challenging and demands a higher level of mathematical maturity and commitment from students.
Exam Length and Format Differences
While both exams share a similar structure, the AP Calculus BC exam is longer and covers a broader range of topics. Here are the key differences:
- Exam Length: The AP Calculus BC exam covers additional topics compared to the AP Calculus AB exam.
- Sub-scores: The AP Calculus BC exam provides an AB sub-score, which reflects a student’s performance on the portion of the exam that covers the AB material. This sub-score can be useful for college placement and credit.
Ideal Student Profiles
The ideal student profiles for each course differ based on the course content and difficulty:
- AP Calculus AB: Suitable for students who have a solid foundation in algebra, geometry, and precalculus and are seeking a paced introduction to calculus. These students typically have strong mathematical skills but may prefer a more gradual and focused approach to learning calculus.
- AP Calculus BC: Ideal for students who have excelled in previous math courses and are ready for a fast-paced, challenging curriculum that covers both introductory and more advanced calculus topics. These students often have a strong interest in pursuing STEM fields and are looking for a comprehensive calculus course that prepares them for higher-level mathematics.
Benefits and Challenges
The benefits and challenges of each course also vary:
- AP Calculus AB:
- Benefits: Solid foundation, manageable pace
- Challenges: Less challenging for advanced students
- AP Calculus BC:
- Benefits: Comprehensive understanding, beneficial for STEM fields
- Challenges: Demanding workload, requires strong math background
Choosing Between AP Calculus AB and BC
Deciding between AP Calculus AB and BC can be a significant decision in your academic journey. Here are some key factors to consider when making your choice:
1. Content Coverage
AP Calculus AB: Focuses on fundamental calculus concepts such as limits, derivatives, and integrals. It provides a solid foundation in calculus principles.
AP Calculus BC: Covers all the material in AP Calculus AB and extends into more advanced topics such as sequences and series, parametric, polar, and vector functions, and advanced integration techniques.
2. Difficulty Level
AP Calculus AB: Generally considered more manageable for students who prefer a slower-paced introduction to calculus.
AP Calculus BC: Offers a faster-paced, more challenging curriculum suited for students with strong mathematical abilities and a willingness to tackle advanced material.
3. Academic Goals
Consider your long-term academic and career aspirations. If you plan to pursue STEM fields or advanced studies in mathematics, AP Calculus BC may provide more comprehensive preparation.
4. Personal Preferences
Reflect on your learning style and preferences. Are you comfortable with a faster-paced course, or do you prefer a more gradual approach? Choose the course that aligns best with your strengths and preferences.
5. Consultation and Guidance
Seek advice from teachers, advisors, and peers who can provide insights based on your individual strengths and goals.
College Credit and Placement: AB vs. BC
How Colleges View AP Calculus AB
- College Credit: Many colleges and universities offer credit for a qualifying score on the AP Calculus AB exam. The specific score required for credit varies by institution.
- Placement: Depending on the college, a high score on the AP Calculus AB exam may allow you to place into a higher-level calculus course, such as Calculus II.
How Colleges View AP Calculus BC
- College Credit: AP Calculus BC typically offers more college credit compared to AP Calculus AB. A high score on the BC exam may earn you credit equivalent to multiple semesters of college calculus.
- Placement: A strong performance on the AP Calculus BC exam can often result in placement into advanced mathematics courses beyond Calculus II
Considerations for Choosing Between AB and BC
- College Requirements: Research the credit and placement policies of the colleges you are interested in attending. Some institutions may require a specific score on either the AB or BC exam for credit or placement, for example at the University of Michigan College of Engineering, getting a 5 on AB Calculus gets you 4 credit hours, but getting a 5 on BC Calculus gets you 8 credit hours.
- Mathematical Goals: Consider your mathematical goals and how AP Calculus fits into your academic plan. If you aim to pursue a STEM major or advanced math courses, AP Calculus BC may provide more comprehensive preparation.
- Personal Preference: Ultimately, choose the course that aligns best with your strengths, interests, and academic goals. You can use this tool to find colleges that offer credit or placement for AP scores.
Preparing for the AP Calculus Exams
To excel on the AP Calculus AB or BC exams, follow these key tips:
1. Know the Exam Format
Understand the structure and types of questions on the exam by reviewing past papers.
2. Master Core Concepts
Focus on understanding fundamental calculus concepts, including limits, derivatives, and integrals.
3. Practice Regularly
Dedicate time to solving a variety of problems from textbooks, practice exams, and online resources.
4. Use Review Materials
Supplement your learning with review books, study guides, and online resources.
5. Utilize Technology
Familiarize yourself with graphing calculators and use them to visualize concepts and solve problems.
6. Take Practice Exams
Take timed practice exams to simulate the test environment and identify areas for improvement.
7. Review and Reflect
Analyze your performance on practice exams and adjust your study plan accordingly.
8. Stay Confident
Stay confident in your preparation and manage test anxiety with relaxation techniques.
Conclusion: Choosing Your Path in AP Calculus
Deciding between AP Calculus AB and BC is a significant step in your academic journey. Consider your goals, abilities, and preferences carefully:
Know Your Goals
Consider your long-term academic and career aspirations.
Assess Your Abilities
Evaluate your mathematical strengths and learning style.
Seek Guidance
Consult with teachers, advisors, and peers for advice.
Embrace the Challenge
Approach either course with enthusiasm and a willingness to learn.
Remember, It’s Your Journey
Focus on making the most of your learning experience.
Still confused? Connect with us and take an expert advice!
Frequently Asked Questions (FRQs) :
Q. Which is harder AP Calculus AB or BC?
In reality, AP Calculus BC is harder than AP Calculus AB. AP Calculus BC not only covers all the topics in AP Calculus AB but also includes additional advanced topics such as sequences and series, parametric equations, polar coordinates, and more complex integration techniques. This means that AP Calculus BC demands a higher level of mathematical understanding and a faster pace of learning. If you have a strong foundation in math and are prepared for a challenging course, AP Calculus BC offers a more comprehensive and rigorous curriculum. However, if you prefer a more gradual introduction to calculus, AP Calculus AB might be the better choice.
Q. Which Physics course can I take with AP Calculus AB or BC?
Although both AP Physics 1 and AP Physics C can be taken, however for someone taking Physics for the first time, AP Physics 1 is an apt choice. It is an Algebra based course. Taking AP Calculus AB and AP Physics 1 together is a pretty popular choice.



