Conquering calculus? AP Calculus BC might be the key that reveals all about mathematical possibilities. This rigorous course gets into the fascinating world of derivatives, integrals, and their applications, preparing you for college-level mathematics and potentially earning college credit. But before you take on, here’s a comprehensive guide to equip you for AP Calculus BC success.
What is AP Calculus BC?
Offered by the College Board, AP Calculus BC is an Advanced Placement (AP) program. It’s a year-long calculus course designed to be equivalent to a full year of college calculus. Compared to its counterpart, AP Calculus AB ,AP Calculus BC covers a broader range of topics, venturing deeper into theoretical concepts and applications.
What Will You Learn in AP Calculus BC?
The AP Calculus BC curriculum revolves around several key areas, providing a rich and diverse learning experience:
Differential Calculus:
This branch focuses on the concept of limits and derivatives. You’ll learn how to find the instantaneous rate of change of a function (the derivative), analyse its behaviour using derivative tests (increasing/decreasing intervals, concavity), and apply it to solve optimization problems (maximum/minimum values).
Limits:
You’ll explore the concept of limits, the cornerstone of calculus. Understanding how functions approach specific values as their inputs get closer and closer is crucial for grasping derivatives and other advanced topics.
Derivatives:
Derivatives are the heart of differential calculus. You’ll learn how to find the derivative of various functions (polynomial, exponential, logarithmic, trigonometric, etc.) using differentiation rules and apply them to solve real-world problems involving motion, rates of change, and optimization.
Applications of Derivatives:
This section puts your newfound knowledge of derivatives to work. You’ll learn how to analyse motion problems (related rates, extrema), solve optimization problems in various contexts (business, economics, physics), and delve into applications in physics, engineering, and economics.
Integral Calculus:
Here, you’ll explore the concept of finding the accumulated area or volume under a curve (the integral). You’ll master techniques like the Fundamental Theorem of Calculus, a powerful tool that connects differentiation and integration. You’ll also apply them to solve definite and indefinite integrals and understand their connection with derivatives.
Indefinite Integrals:
You’ll delve into the concept of finding the antiderivative of a function (the indefinite integral). This provides the foundation for solving a wide range of calculus problems.
Definite Integrals:
Here, you’ll learn how to calculate the definite integral of a function, representing the accumulated area or volume under the curve over a specific interval.
The Fundamental Theorem of Calculus:
This theorem bridges the gap between differentiation and integration, allowing you to efficiently solve definite integral problems using the antiderivatives you found earlier.
Applications of Calculus:
This section builds upon your understanding of derivatives and integrals to model real-world phenomena using calculus. You’ll learn how to:
- Solve motion problems involving velocity and acceleration using definite integrals.
- Analyse work and energy concepts in physics using definite integrals.
- Apply calculus to solve economic problems involving marginal cost, marginal revenue, and consumer surplus.
Sequences and Series:
This unit explores infinite sequences and series, their convergence or divergence (whether they approach a finite value or not), and their applications in representing functions and solving mathematical problems.
Sequences:
You’ll learn how to analyse sequences, understand their convergence properties, and explore their applications in various mathematical contexts.
Series:
This section delves into infinite series, formed by summing the terms of an infinite sequence. You’ll learn convergence tests (ratio test, root test, etc.) to determine whether a series converges to a finite value or diverges to positive or negative infinity.
Lower grade courses like AP Precalculus can help in building fundamentals.
Here are some additional topics you’ll encounter in AP Calculus BC that aren’t covered in AB:
Parametric, polar, and vector calculus:
These specialised branches of calculus extend the concepts of derivatives and integrals to more complex functions and geometric shapes.
Applications of derivatives in physics and engineering (work, fluid flow):
You’ll explore how to apply derivatives to solve advanced physics and engineering problems involving work, fluid flow, and other concepts.
Improper integrals:
These integrals represent the limit of a definite integral as one or both of its bounds approach positive or negative infinity.
Taylor Series and infinite series convergence tests:
Taylor Series are powerful tools for representing functions as infinite series of polynomials. You’ll learn how to construct Taylor Series for various functions and explore different convergence tests for infinite series.
Wondering which one to take? Read this blog to guide you on AP Calculus AB or BC – Which one to take?
Paper Structure and Scoring of AP Calculus BC Exam
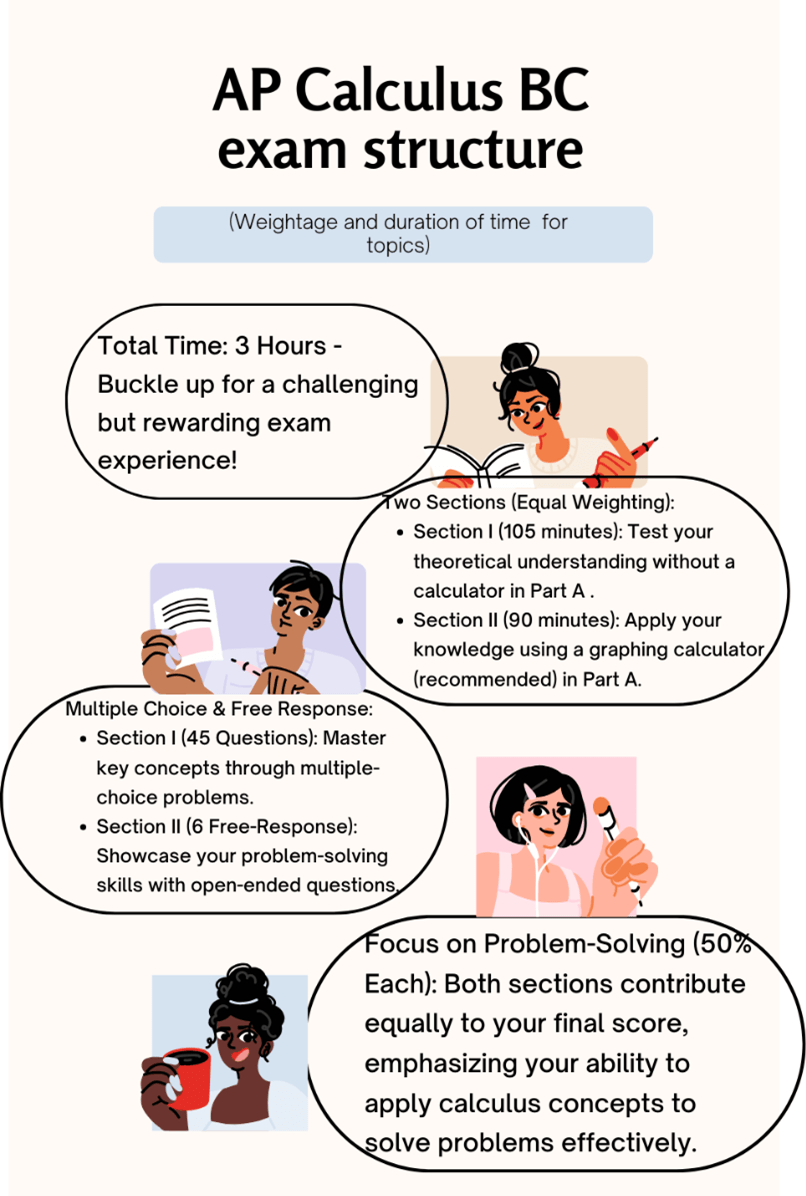
The AP Calculus BC exam is a three-hour long, multiple-choice and FRQs exam, divided into two sections:
Section I (50% of exam weight):
Duration: 1 hour 45 minutes (Part A: 60 minutes; Part B: 45 minutes)
Calculator Use: Not allowed (Part A); Allowed (Part B)
Question Types: This section focuses on theoretical understanding and problem-solving without a calculator. It consists of 45 multiple-choice questions that assess your grasp of:
- Limits and continuity
- Derivatives (finding derivatives, applying derivative tests for increasing/decreasing intervals and concavity, using derivatives in applications)
- Applications of derivatives in various contexts (related rates, optimization, motion)
- Sequences and Series (convergence/divergence)
Section II (50% of exam weight):
Duration: 1 hour 30 minutes (Part A: 30 minutes ; Part B: 60 minutes)
Question Types: 6 Free-response questions that involve:
- Derivatives (finding derivatives, using derivatives in applications)
- Integrals (definite and indefinite integrals, applications of integrals)
- Sequences and Series (convergence/divergence)
It’s further divided into two parts:
Part A (16.7% of exam weight),
Duration: 30 minutes,
Number of Questions: 2
Calculator use: Allowed
Part B (33.3% of exam weight),
Duration: 60 minutes,
Number of Questions: 4
Calculator use: Not allowed
These questions assess your ability to:
- Apply differential and integral calculus concepts to solve problems
- Demonstrate analytical thinking and problem-solving skills
- Communicate your solutions clearly and concisely
| Sections | Duration
(minutes) |
Question Type | Number of questions asked | Calculator use | Question types |
| Section-I | 60 | Multiple choice questions
Part A:
|
30 |
Not allowed |
Limits, Derivatives (concepts & applications), Sequences & Series (convergence/divergence) |
| 45 |
Part B: |
15 |
Allowed (graphing calculator recommended) | ||
| Section- II | 60 | Free response questions
Part A: |
2 |
Allowed (graphing calculator recommended) | Part A (16.7% of Exam Weight)
Duration: 60 minutes Question Types: Free-Response (apply differential & integral calculus, demonstrate problem-solving & communication skills) |
| 30 |
Part B:
|
4 |
Not allowed |
Part B (33.3% of Exam Weight)
Duration: 30 minutes Question Types: Derivatives (concepts & applications), Integrals, Sequences & Series (convergence/divergence)
|
To know about the course and exam description, download sample papers and more, click here
Exam Weighting for the Multiple-Choice Section of the AP Exam
| Units | Weightage |
| Unit 1: Limits and Continuity | 4–7% |
| Unit 2: Differentiation: Definition and
Basic Derivative Rules |
4–7%
|
| Unit 3: Differentiation: Composite,
Implicit, and Inverse Functions |
4–7%
|
| Unit 4: Contextual Applications of
Differentiation |
6–9%
|
| Unit 5: Applying Derivatives to Analyse
Functions |
8–11%
|
| Unit 6: Integration and Accumulation
of Change |
17–20%
|
| Unit 7: Differential Equations | 6–9%
|
| Unit 8: Applications of Integration | 6–9%
|
| Unit 9: Parametric Equations, Polar
Coordinates, and Vector-Valued Functions
|
11–12%
|
| Unit 10: Infinite Sequences and Series
|
17–18%
|
Practice and Resources for AP Calculus BC
Following up on the previous section’s emphasis on preparation, let’s delve deeper into practical strategies to hone your skills and explore valuable resources for AP Calculus BC:
- Form a Study Group: Collaborating with classmates is a powerful way to solidify concepts. Discussing problem-solving approaches, explaining concepts to each other, and identifying areas needing improvement can significantly enhance your understanding.
- Practice regularly: Calculus is a subject best learned by doing. Regularly practise solving problems from your textbook, past AP exams (available on the College Board Website ), and online resources.
- Focus on Problem-Solving Strategies: Don’t just memorise formulas. Aim to understand the underlying concepts and develop a strategic approach to tackling different problem types.
- Time Management is Key: The AP Calculus BC exam has time constraints. Practice timed problem-solving sessions to build your speed and accuracy under pressure.
- Online Resources: The internet is a treasure trove of calculus learning materials, you can check our own platform Tutoringmaphy .
- Khan Academy: A fantastic free resource with video tutorials, practice problems, and personalised learning paths.
- MIT Open Courseware: Access video lectures, course materials, and practice problems from MIT’s Calculus Course .
Additional Tips
Remember, the key to success in AP Calculus BC lies in a combination of dedication, the right approach, and utilising the available resources. Here are some additional tips to help you on your journey:
- Don’t Be hesitant to Ask for Help: If you’re struggling with a concept, don’t hesitate to seek help from your teacher, classmates, or online tutors.
- Stay Motivated: The AP Calculus BC course can be challenging. Set achievable goals, celebrate your progress, and reward yourself for your hard work
- Develop a Growth Mindset: View challenges as opportunities to learn and grow. Embrace mistakes as stepping stones to improvement.
Frequently Asked Questions (FAQs)
Q: Is AP Calculus BC hard?
A: AP Calculus BC is a demanding course, but with dedication, a strong foundation in maths, and the right resources, it’s achievable.
Interested to know about the hardest AP exams? Check out this two part blog: A guide to the most difficult AP Exams Part 1 and Part 2
Q: What are the benefits of taking AP Calculus BC?
A: There are several advantages:
- College Credit: A high score on the AP exam can earn you college credit, potentially reducing your college workload. If you are interested to know more about credits, checkout How are AP and College credits related?
- Strong Math Foundation: The course provides a solid foundation for further mathematics studies in college.
- Problem-Solving Skills: Calculus hones your problem-solving skills, valuable for various academic disciplines and careers.
- College Preparation: It prepares you for the rigour of college-level mathematics courses.
Q: How long is the AP Calculus BC exam?
A: The AP Calculus BC exam is a three-hour-long test (excluding 15 min break) designed to assess your understanding of the course curriculum. It’s divided into two sections:
Section I (50% of exam weight): MCQs
Duration: 1 hour 45 minutes (Part A: 60 minutes; Part B: 45 minutes)
Calculator Use: Not allowed (Part A); Allowed (Part B)
Section II (50% of exam weight): FRQs
Duration: 1 hour 30 minutes (Part A: 30 minutes ; Part B: 60 minutes)
Q: Is there a formula sheet for AP Calculus BC?
A: No, there is no formula sheet provided on the AP Calculus BC exam. You’re expected to memorise and understand the essential formulas for derivatives, integrals, and other relevant concepts. However, the exam focuses more on applying these formulas to solve problems rather than simply recalling them.
Q: What is the difference between AP Calculus BC and AP Calculus AB?
A: Both AP Calculus AB and AP Calculus BC cover differential and integral calculus, but AP Calculus BC goes deeper into the subject matter and introduces additional topics. Here’s a breakdown of the key differences:
Scope:
AP Calculus AB: Covers foundational concepts of differentiation and integration, focusing on applications in motion, kinematics, and basic optimization problems.
AP Calculus BC: Extends beyond AP Calculus AB, exploring applications in physics, engineering, and economics. It also delves into parametric, polar, and vector calculus, improper integrals, and Taylor Series.
Depth: AP Calculus BC goes deeper into the theoretical aspects of calculus compared to AP Calculus AB.
Checkout this article to know more about AP Calculus AB.
Q: What resources can help me succeed in AP Calculus BC?
A: There are numerous resources available, including textbooks, online courses, practice problems from past exams, websites like Tutoringmaphy, Khan Academy and MIT OpenCourseware, and the official AP resources provided by the College Board.
In conclusion, AP Calculus BC presents a challenging yet rewarding academic journey. By equipping yourself with the necessary knowledge, resources, and a strategic approach, you can conquer this course and unlock a deeper understanding of the fascinating world of calculus.
Next: Planning to take AP Physics C Mechanics as well? You can check out AP Physics C Mechanics: Key Points You Need to Know



