I. Introduction: Brief overview of AP Precalculus Unit 1
Alright, in AP Precalculus Unit 1, we’re getting into some basic math stuff. This Unit requires about 6-8 weeks of prep time and covers 30-40% of AP Exam weightage. We’re talking functions, polynomials, rational functions, and more. This unit is like the starting line for digging deeper into precalculus and beyond. We’re laying down the basics here so we can tackle the tougher stuff later on. By the way, here’s the complete curriculum.
You can also book an experienced and affordable private online AP Precalculus tutor.
Basically, we’re going on a bit of a math journey. We’re going to check out all these key concepts, understand them inside and out. It’s like building a solid foundation for a big math journey ahead. As we go through each topic one by one, students get a good grip on the fundamental math ideas that they’ll need for the more advanced stuff down the road. Further Units of AP Precalculus, Unit 2 and Unit 3, are build on the learning from this Unit.
II. Functions
Functions are like the building blocks of math, giving us a way to make sense of how different things relate to each other. In AP Precalculus, we’re really getting into functions, looking at them from all angles. We’ll start with the basics in Unit 1 and then move on to more complex stuff in later units, like how things change over time. Going further, this is also a very important topic for Calculus BC. Here is a detailed overview.
1.1 Change in Tandem
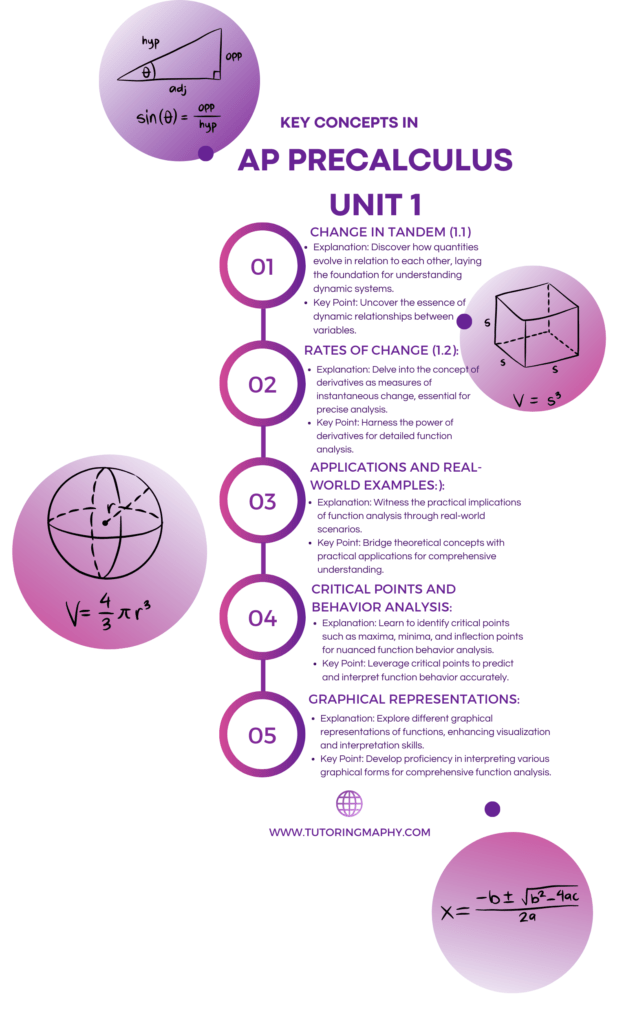
1.1 change in tandem sets the stage for understanding functions by examining how quantities change in tandem/relation. Here, students explore the fundamental properties of functions and their representations. Through the analysis of linear, quadratic, polynomial, exponential, and logarithmic functions, students begin to understand the dynamic nature of relationships between variables. Unit 1 introduces students to the concept of change and its realization within the purview of functions.
1.2 Rates of Change
1.2 the focus shifts to rates of change, exploring how functions change over time. Students look into the concept of the derivative as a measure of instantaneous rate of change at a specific point. Through various techniques, students learn to analyze the behavior of functions with greater precision, learning about their slopes, concavity, and critical points. This unit equips students with the tools to quantify and interpret changes in various contexts, including the word problems.
1.3 Rates of Change in Linear and Quadratic Functions
1.3 narrows the focus to rates of change of linear and quadratic functions. Students analyze the slopes and concavities of these functions, gaining a deeper understanding of how changes in one variable affect changes in another. Through graphical analysis and algebraic manipulation, students learn patterns and relationships that allow them to make predictions and solve real-world problems. This unit provides valuable insights, preparing students for more advanced studies in calculus.
As students progress through these units, they not only develop a deeper understanding of functions but also refine their analytical skills and problem-solving abilities. By mastering the concepts presented in each unit, students build a solid foundation for success in AP Precalculus.
II. Polynomials
Polynomials are widely used mathematical expressions that find applications across various fields, from physics to economics. In AP Precalculus, the study of polynomials is about their properties, behavior, and applications. Let’s explore how polynomials are examined and understood in the context of different units, focusing on their rates of change, complex zeros, and end behavior.
1.4 Polynomial Functions and Rates of Change
In 1.4, the exploration extends to polynomial functions, where students analyze how these functions relate. By examining the slopes and concavities of polynomial functions, students gain insights into their rates of change and behavior. Understanding the rates of change of polynomial functions allows students to make predictions, solve problems, and interpret real-world phenomena with greater precision.
1.5 Polynomial Functions and Complex Zeros
Complex zeros are an essential aspect of polynomial functions, representing the points at which the function crosses the x-axis in the complex plane. In 1.5, students explore the relationship between polynomial functions and their complex zeros. By employing techniques such as synthetic division and the fundamental theorem of algebra, students learn to identify and analyze complex zeros, gaining a further understanding of the behavior and structure of polynomial functions.
1.6 Polynomial Functions and End Behavior
The end behavior of polynomial functions provides valuable insights into their long-term behavior as x approaches positive or negative infinity. In 1.6, students investigate the end behavior of polynomial functions, distinguishing patterns and relationships that governs their asymptotic behavior. By analyzing the leading term and degree of polynomial functions, students can determine their end behavior and make predictions about their graphs.
IV. Rational Functions
Rational functions are essential mathematical constructs. In AP Precalculus, the study of rational functions delves into their properties, behavior, and application, focusing on their end behavior, zeros, vertical asymptotes, and holes. Let’s explore.
1.7 Rational Functions and End Behavior
In 1.7, students explore the end behavior of rational functions, gaining insights into their long-term behavior as x approaches positive or negative infinity. By analyzing the leading terms of the numerator and denominator, students can determine the end behavior of rational functions and make predictions about their graphical representations.
1.8 Rational Functions and Zeros
Zeros are points of rational functions, representing the values of x for which the function equals zero. In 1.8, students investigate the relationship between rational functions and their zeros, employing techniques such as factoring to identify and analyze zeros. Understanding the distribution and properties of zeros allows students to sketch accurate graphs of rational functions and interpret their behavior
1.9 Rational Functions and Vertical Asymptotes
Vertical asymptotes are vertical lines that the graph of a rational function approaches but never crosses. In 1.9, students explore the relationship between rational functions and their vertical asymptotes, identifying the values of x that result in division by zero. By analyzing the behavior of rational functions near vertical asymptotes, students gain insights into their graphical representations and understand how these functions behave as x approaches certain values.
Curious about reliable resources of AP Precalculus? Checkout Top 10 books for AP Precalculus
1.10 Rational Functions and Holes
Holes, also known as removable discontinuities, are points where a rational function is undefined but can be “filled in” to make the function continuous. In 1.10, students learn to identify and analyze holes in rational functions, understanding their causes and effects on the function’s behavior.
V. Transformation and Modeling
In AP Precalculus, the study of transformation and modeling involves exploring how functions can be manipulated and applied to model various phenomena. Let’s delve into how transformation and modeling are examined – in relation to shifting graphs, dilation, reflection and so on.
Looking for extra practice? Check out our collection of free downloadable AP Precalculus worksheets with solutions.
1.12 Transformations of Functions
In 1.12 students explore how functions can be transformed through translations, reflections, stretches, and compressions. By applying these transformations to basic functions such as linear, quadratic, and exponential functions, students gain insights into how changes in parameters affect the shape, position, and behavior of graphs. Understanding transformations allows students to manipulate functions to fit specific criteria or model real-world scenarios accurately.
1.13 Function Model Selection and Assumption Articulation
Selecting an appropriate function model is crucial for accurately representing a mathematical phenomenon. 1.13, students learn to articulate assumptions and select function models that best fit the data or situation at hand. By considering factors such as domain, range, behavior, and context, students can identify the most suitable function model and justify their choice based on mathematical principles and real-world constraints. Articulating assumptions ensures that the chosen function model accurately reflects the relationships and provides meaningful insights for analysis and prediction.
1.14 Function Model Construction and Application
Constructing function models involves using mathematical techniques to fit data or describe phenomena accurately. In 1.14, students learn to construct function models based on given data or specifications. By employing regression analysis, curve fitting, and other modeling techniques, students develop function models that capture the important features of the data and helps in analysis and prediction.
VI. AP Precalculus Unit 1 Practice and tutoring
Running out of practice questions on AP Precalculus Unit 1? Check out
Are you also preparing for SAT? Familiarize yourself with The New Digital SAT Format



